A triangle is a special right triangle with some very special characteristics If you have a degree triangle, you can find a missing side length without using the Pythagorean theorem!Corresponding to opposite angle measures, the ratio of the side lengths of every triangle is 1√32;Start studying Combo with Special Right Triangles and and 1 other Learn vocabulary, terms, and more with flashcards, games, and other study tools

Test Prep Thursday Special Right Triangles Mathnasium
Special right triangles 30 60 90 formula
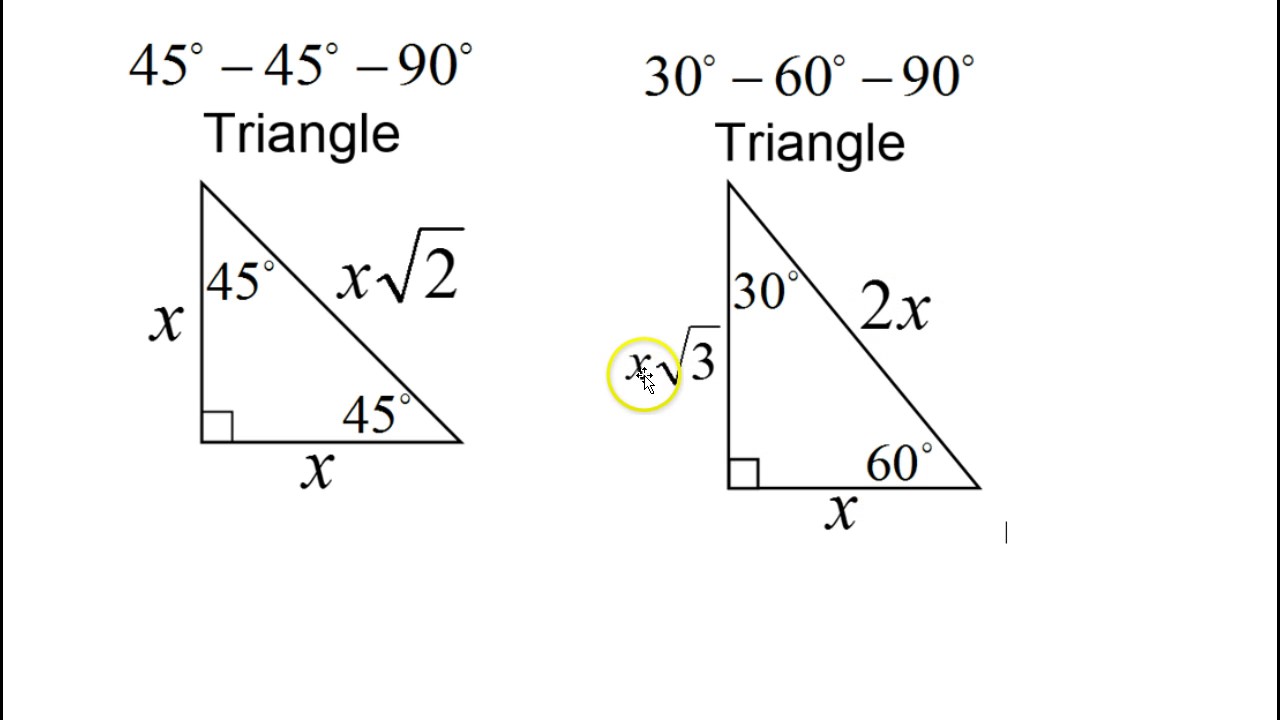
Special right triangles 30 60 90 formula-Learn shortcut ratios for the side lengths of two common right triangles 45°45°90° and 30°60°90° triangles The ratios come straight from the Pythagorean theoremCheck out this tutorial to learn about triangles!



Triangle 30 60 90 Clipart Etc
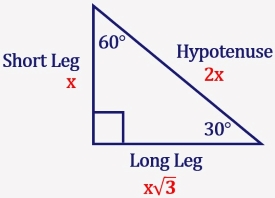
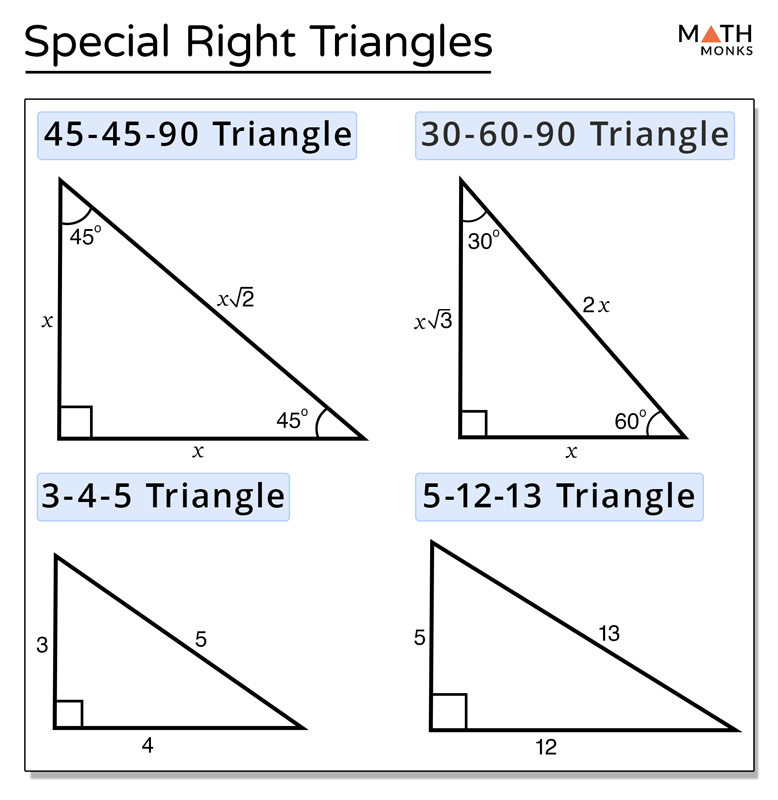
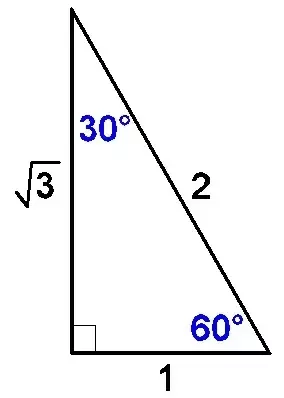
Special Right Triangles 30°60°90° triangle The 30°60°90° refers to the angle measurements in degrees of this type of special right triangle In this type of right triangle, the sides corresponding to the angles 30°60°90° follow a ratio of 1√ 32 Thus, in this type of triangle, if the length of one side and the side's 45° 45° 90° Triangles A right triangle with two sides of equal lengths is a 45° 45° 90° triangle The length of the sides are in the ratio of 11 √2 Leg length = 1/2 hypotenuse√2 Hypotenuse = leg√2 30° 60° 90° Triangles Hypotenuse is always opposite the right angle Short Leg is opposite the 30 angleThe 30°–60°–90° triangle is the only right triangle whose angles are in an arithmetic progression The proof of this fact is simple and follows on from the fact that if α, α δ, α 2δ are the angles in the progression then the sum of the angles 3α 3δ =
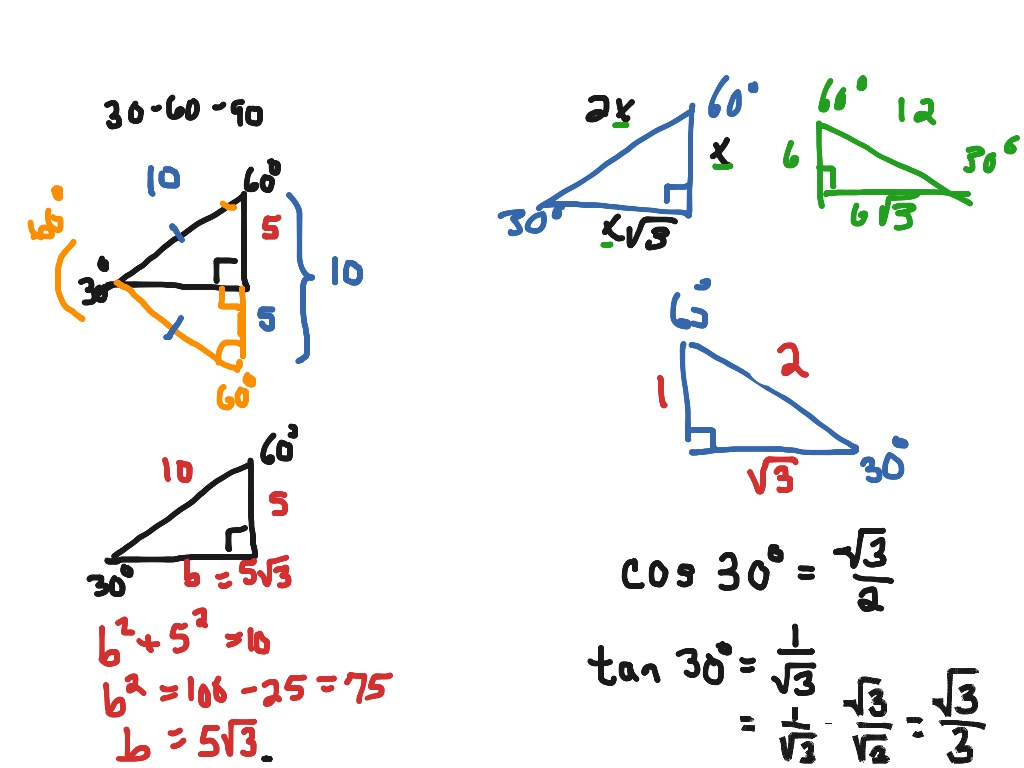
Here, we will look at the triangle We also have lessons on other special right triangles Triangles The triangle is one example of a special right triangle It is right triangle whose angles are 30°, 60° and 90° The lengths of the sides of a triangle are in the ratio of 1√32In plain language, the hypotenuse is twice as long as the shortest leg (opposite the 30 degree angle), and the longest leg (opposite the 60 degree angle) is √3 times longer thanBig Idea Students discover the patterns involved in a triangle Use congruence and similarity criteria for triangles to solve problems and to prove relationships in geometric figures Make sense of problems and persevere in solving them Reason abstractly and quantitatively
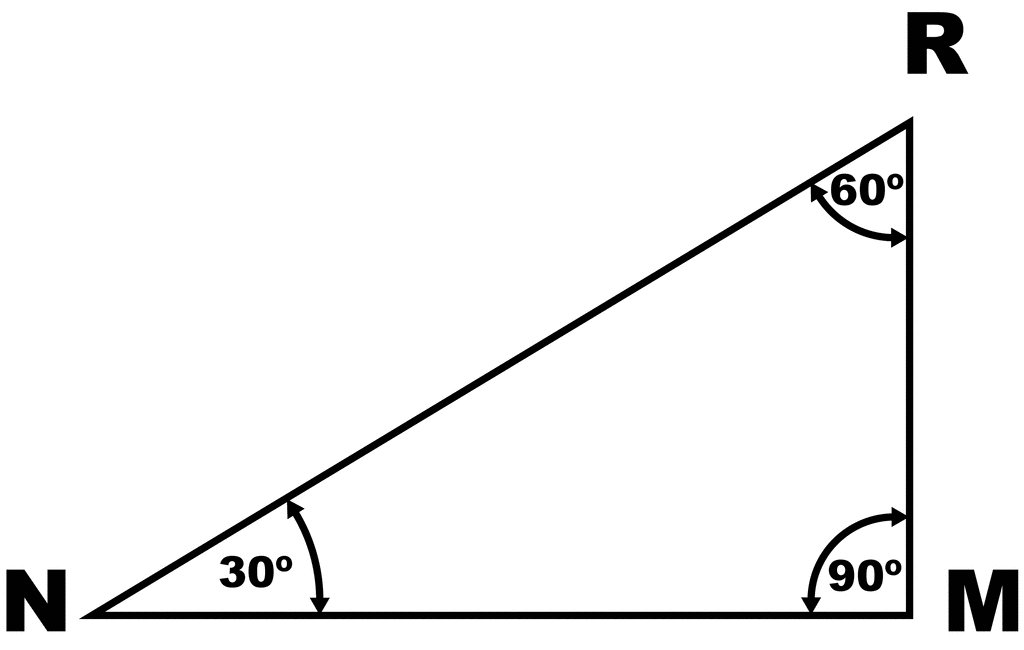
A right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in an easytoremember ratio 1 √3 3 2 That is to say, the hypotenuse is twice as long as the shorter leg, and 30 30° 60° 90° The triangle is based on an equilateral triangle with sides of 2 units 2 2 2 60 ° 60 ° 31 30° 60° 90° The altitude (also the angle bisector and median) cuts the triangle into two congruent triangles 1 1 30 ° 30 ° 2 2 2 60 ° 60 ° 32 30° 60° 90° This creates the triangle with a hypotenuse a short leg and a long leg hypotenuse ShortSpecial right triangles (practice) Khan Academy Use the Pythagorean theorem to discover patterns in 30°60°90° and 45°45°90° triangles Use the Pythagorean theorem to discover patterns in 30°60°90° and 45°45°90° triangles If you're seeing this message, it means we're having trouble loading external resources on our website




Special Right Triangles 30 60 90 Worksheet



Special Right Triangle 30 60 90 Mathondemand Com
Triangle Practice Name_____ ID 1 Date_____ Period____ ©v j2o0c1x5w UKVuVt_at iSGoMfttwPaHrGex rLpLeCkQ l ^AullN Zr\iSgqhotksV vrOeXsWesrWvKe`d\1Find the missing side lengths Leave your answers as radicals in simplest form 1) 12 m n 30° 2) 72 ba 30° 3) x y 5 60° 4) x 133y 60° 5) 23 u v 60° 6) m n63Right triangle calculator, 30 60 90 formula, 45 triangle, special area, unit circle calculatorSpecial Triangles Right Triangles FoldableThis foldable explains in an easy way special right triangles theorems Theorem and Theorem Your students will be able to visualize the formulas to find sides lengths of special right triangles and use them to solve problemsThis is a gre




30 60 90 Right Triangle Side Ratios Expii




Test Prep Thursday Special Right Triangles Mathnasium
Special Right Triangles Date_____ Period____ Find the missing side lengths Leave your answers as radicals in simplest form 1) a 2 2 b 45° 2) 4 x y 45° 3) x y 3 2 2 45° 4) x y 3 2 45° 5) 6 x y 45° 6) 2 6 y x 45° 7) 16 x y 60° 8) u v 2 30°1 Special Right Triangles (, ) Created by user Linda Gregory I use this activity to have my students discover the relationships between the sides on and triangles Materials required rulers Activity Download Special_Right_Trianglesdocx Activity Answer Key 30 6090 triangles 1 Special Right Triangles 30 – 60 – 90 Triangles 2 Special Right Triangles Directions As you view this presentation, take notes and work out the practice problems When you get to the practice problem screens, complete the step in your notebook before continuing to the next slide 3



Special Right Triangles Definition Formula Examples




30 60 90 Triangle Explanation Examples
The triangle name explains the three internal angles These triangle likewise have side length connections that can easily remember The photo below programs all angle and side length partnerships for the and triangles An anglebased special right triangle SideBased Special Right Triangles The usual sidebased specialTheorem Application of Pythagoras #short #shortvideo #shortsfeedWhat I want to do in this video is discuss a special class of triangles called triangles and I think you know why they're called this the measures of its angles are 30 degrees 60 degrees and 90 degrees and what we're going to prove in this video this tends to be a very useful result at least for a lot of what you see in a geometry class and then later on in trigonometry class is the




Triangle 30 60 90 Clipart Etc



Special Triangles Hiset Math
The worksheet includes 15 problems, 9 which include a diagram and 6 story problemsSpecial Right Triangles Although all right triangles have special features – trigonometric functions and the Pythagorean theorem The most frequently studied right triangles, the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 trianglesLearn how to solve for the sides in a Special Right Triangle in this free math video tutorial by Mario's Math Tutoring009 What are the Ratios of t




Right Triangles Interactive Notebook Pages Mrs E Teaches Math




Trigonometry Special Right Triangles 30 60 90 Triangle Answers Need To Be In Radical Form Cant Brainly Com
Tahoma Arial Wingdings Bradley Hand ITC Monotype Corsiva Symbol Comic Sans MS Times New Roman Blends 1_Blends Special Right Triangles 45°45°90° Triangles 45°45°90° Triangles 45°45°90° Triangles Special Right Triangles 30°60°90° Triangles 30°60°90° Triangles 30°60°90° Triangles Special Right Triangles Special Right A is a scalene triangle and each side has a different measure Since it's a right triangle, the sides touching the right angle are called the legs of the triangle, it has a long leg and a short leg, and the hypotenuse is the side across from the right angle Slide Topic Special Right Triangles Worksheet Maze Activity 30 60 90 45 45 90 Triangle Worksheet Right Triangle Word Problem Worksheets Worksheet 81 Similarity In Right Triangles Answers Presentation Time 6 minutes File Format PPT File size 34mb Number of Pages 79 slides Publication Date August 19 Open Special Right Triangles Worksheet Maze



30 60 90 Right Triangle How Do I Solve If The Long Leg Is 10 Socratic




30 60 90 Right Triangles Read Geometry Ck 12 Foundation
Start studying P5 Special Right Triangles Learn vocabulary, terms, and more with flashcards, games, and other study toolsA triangle is a right triangle where the three interior angles measure 30 °, 60 °, and 90 ° Right triangles with interior angles are known as special right triangles Special triangles in geometry because of the powerful relationships thatThe triangle is called a special right triangle as the angles of this triangle are in a unique ratio of 123 Here, a right triangle means being any triangle that contains a 90° angle A triangle is a special right triangle that always has



3



Day 16 Test C 9 To 12 Special Right Triangles 30 60 90 45 45 90 Youtube
A triangle with angle degree measures of 30, 60, and 90 is a special right triangle;This video tutorial provides a basic introduction into triangles It explains how to find the value of the missing side of other triangles using thAfter this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangle For this calculation, you would enter your 1 side on the 2nd picture marked and press Solve Triangle Simply enter the one side of the respective right triangle you know located by the picture and press the appropriate Solve




Special Right Triangles 30 60 90 And The 45 45 90 Graphic Organizer Worksheet Special Right Triangle Right Triangle Word Problem Worksheets



30 60 90 Right Triangles Math Trigonometry Trig Triangles Right Triangles Showme
Special Right Triangles () DRAFT 8th University grade 405 times Mathematics 68% average accuracy 3 years ago mollymckee 2 Save Edit Edit In a triangle, Hypotenuse = answer choices Short √2 Short √3 2 Short s Question 7 SURVEY 30 seconds Q The short leg is across from A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one anotherAbout Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us Creators




30 60 90 Triangle Explanation Examples



Special Right Triangles Proof
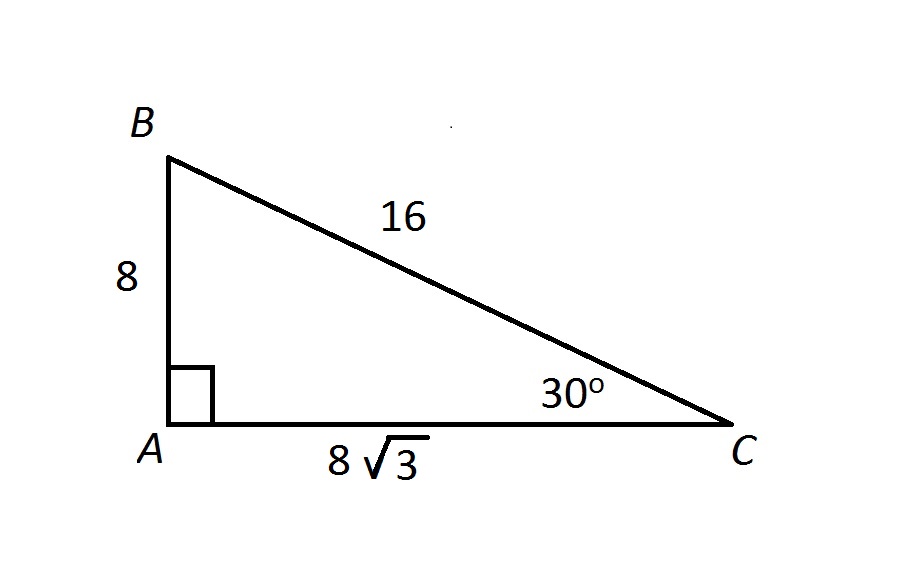
The property is that the lengths of the sides of a triangle are in the ratio 12√3 Thus if you know that the side opposite the 60 degree angle measures 5 inches then then this is √3 times as long as the side opposite the 30 degree so the side opposite the 30 degree angle is 5 /Find the missing sides of the triangles ☐ triangle abc below is Hypotenuse = leg * v2 If you want to know more about another popular right triangles, check out this 30 60 90 triangle tool and the calculator for special right And Triangle Worksheet / Special Right Triangles 45 45 90 Degree And 30 60 90 Geogebra FindSpecial right triangles intro (part 2) Transcript A few more triangle examples and an introduction to triangles Created by Sal Khan Special right triangles Special right triangles intro (part 1) Special right triangles intro (part 2) This is the currently selected item triangle example problem
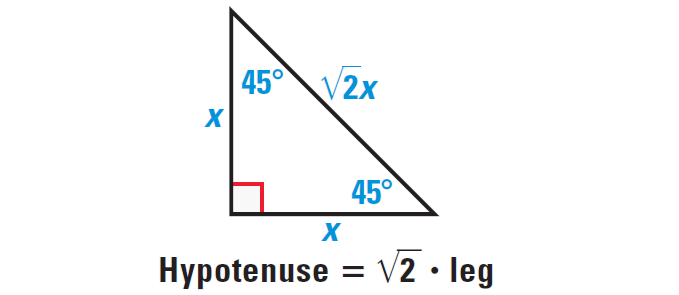



Special Right Triangles




30 60 90 Triangle Calculator Formula Rules
The other most well known special right triangle is the triangle In a triangle, the two nonright angles are 30 and 60 degrees Credit Public Domain A triangle has sides that lie in a ratio 1√32 Knowing these ratios makes it easy to compute the values of the trig functions for angles of 30 degrees (π/6) and 30 60 90 triangle sides If we know the shorter leg length a, we can find out that b = a√3 c = 2a If the longer leg length b is the one parameter given, then a = b√3/3 c = 2b√3/3 For hypotenuse c known, the legs formulas look as follows a = c/2 b = c√3/2 Or simply type your given values and the 30 60 90 triangle calculator will do the rest!Special Right Triangles Use the and triangle relationships to solve for the missing sides Use the answers to reveal the name of the team that Abraham M Saperstein established and sent on the road in 1927




Special Right Triangles Geometry15c



Don T Hate On High School Math Special Right Triangles 30 60 90
There are two "special" right triangles that will continually appear throughout your study of mathematics the 30º60º90º triangle and the 45º45º90º triangle The special nature of these triangles is their ability to yield exact answers instead of decimal approximations when dealing with trigonometric functions This page will deal with the 30º60º90º triangleHow To Find The Missing Sides In A Special Right Triangle Triangle 30 60 90 Clipart Etc 30 60 90 Triangle Equation OnettechnologiesindiacomThis trigonometry video tutorial provides a basic introduction into triangles It explains how to evaluate trigonometric functions such as sine and
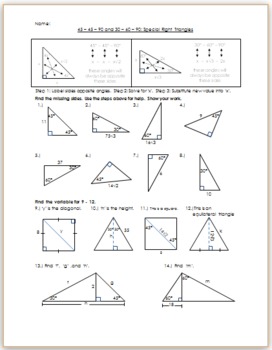



45 45 90 And 30 60 90 Special Right Triangles Practice Hw By Eric Douce




30 60 90 Triangles Special Right Triangle Trigonometry Youtube
This riddle practice worksheet covers 30 60 90 Special Right Triangles by asking students to solve for either the missing leg or for the hypotenuse When they find their answer, they look in the solution box to find the corresponding color to complete the Riddle!Lesson Special Right Triangles Geometry CP Mrs Mongold Make a Frayer Foldable Section 1 Triangle Section 2 Triangle Example for Box 3 Example for box 4 Examples Examples Examples Examples Examples Examples Examples Examples Examples The side of an equilateral triangle measures 21 inches




How To Work With 30 60 90 Degree Triangles Education Is Around




30 60 90 Triangle Identity Gmat Geometry Apex Gmat Blog




Special Right Triangles 30 60 90 Ck 12 Foundation



Math Tutor Berkeley San Francisco Bay Area Mathematics Physics Algebra Geometry Trigonometry Calculus Tutoring Special Right Triangles Mayeri Education




Solving Special Right Triangles Meganmarieulrichs




A Quick Guide To The 30 60 90 Degree Triangle Dummies



2



1




Special Right Triangles



45 45 90 And 30 60 90 Triangles Zona Land Education
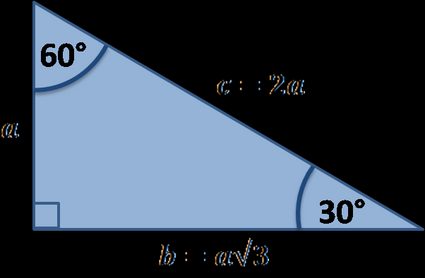



30 60 90 Triangle Calculator Formula Rules




Special Right Triangle 30º 60º 90º A Plus Topper
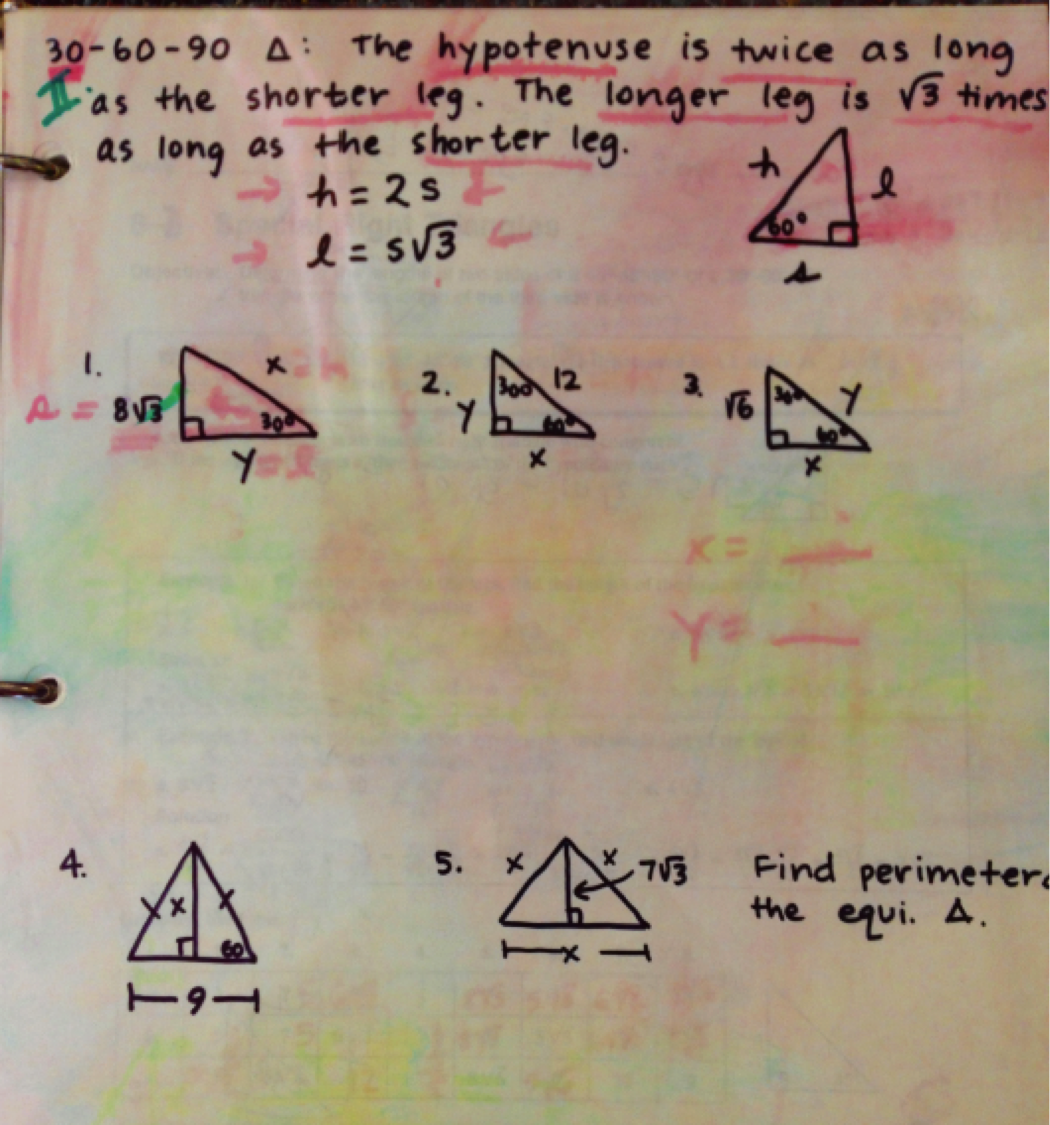



Special Right Triangles Easing The Hurry Syndrome




The Complete Guide To The 30 60 90 Triangle
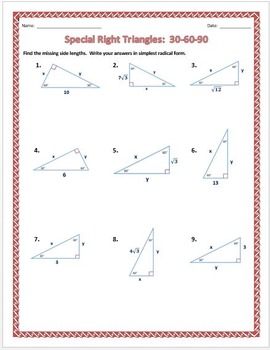



Special Right Triangles 30 60 90 Practice Worksheet By Dr Pepper Lover




Special Right Triangle Ii 30 60 90 3 12 Geometry Survey Name 7 3 Worksheet Special Right Triangles 30 60 90 Period Hypotenuse 2 Short Leg Long Leg Course Hero
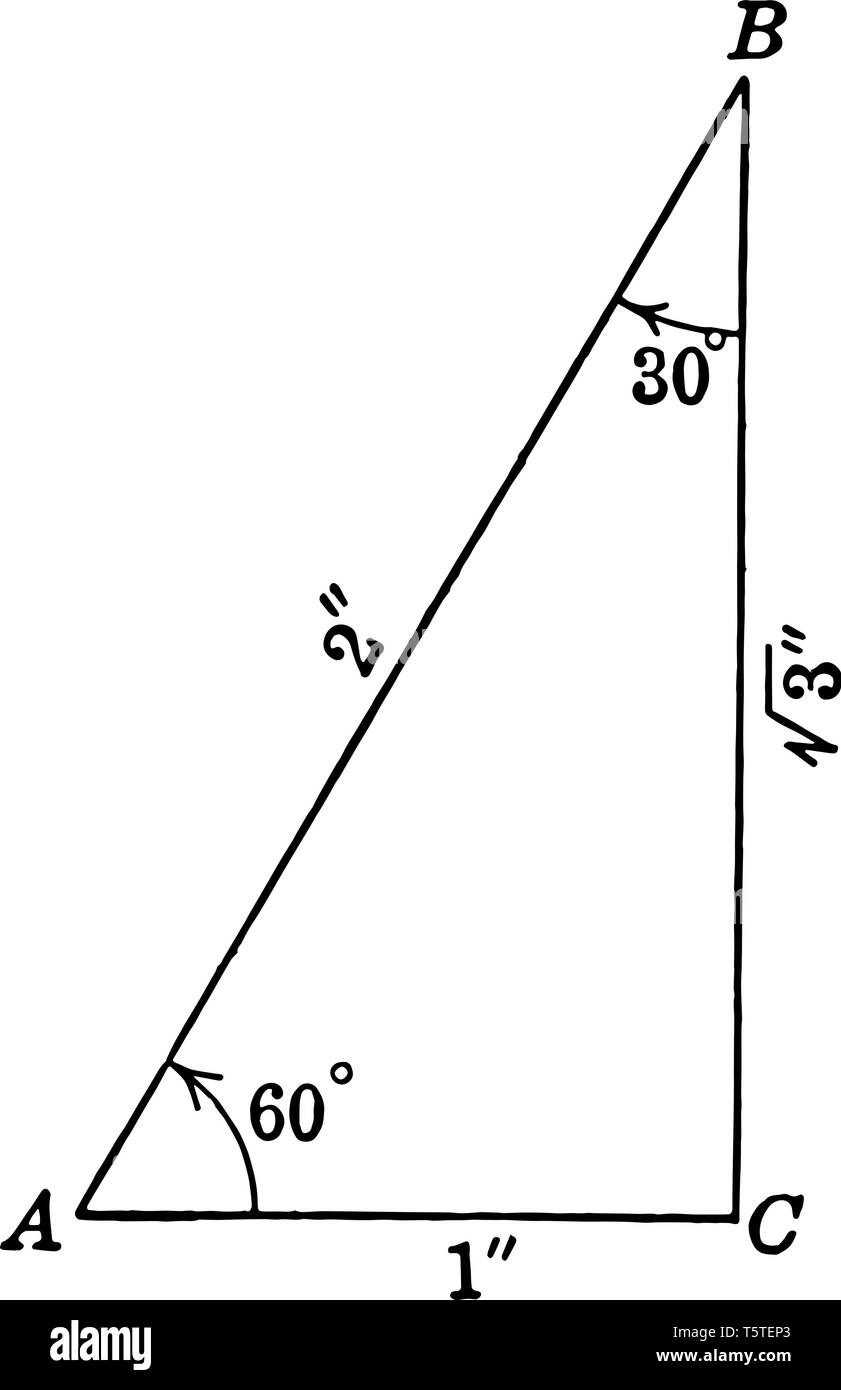



This Image Shows The Special Right Triangle This Image Consists Of Three Angles The Measurement Of The Angles Is 30 60 90 Degrees Vintage Line Draw Stock Vector Image Art Alamy



1
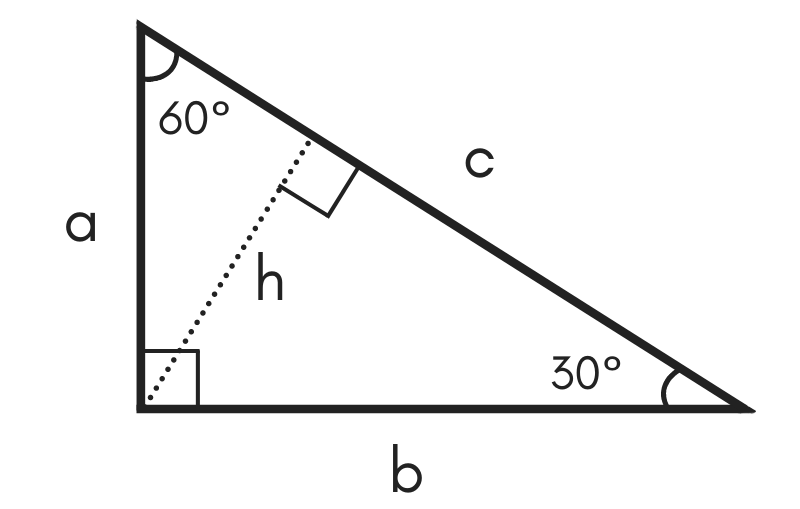



30 60 90 Special Right Triangle Calculator Inch Calculator




Special Right Triangles 30 60 90 Practice Worksheet



30 60 90 Triangles Systry




Special Right Triangles 30 60 90 And 45 45 90 Triangles Youtube
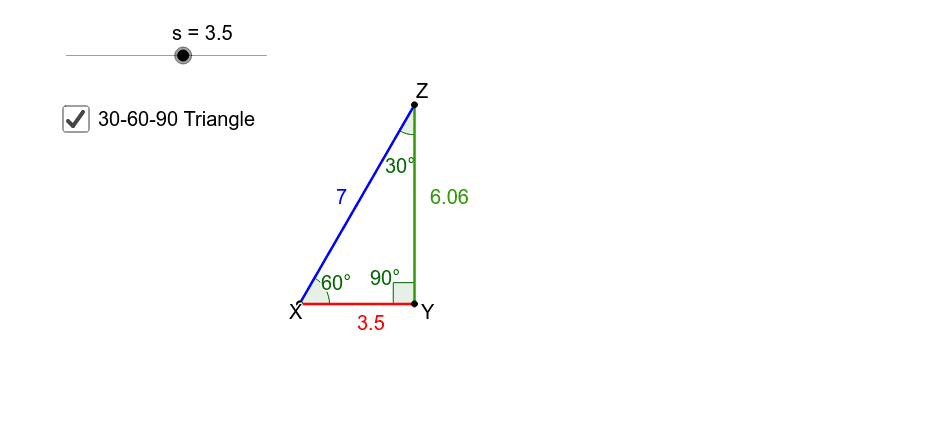



Special Right Triangles 30 60 90 Geogebra




Special Right Triangles Review Article Khan Academy
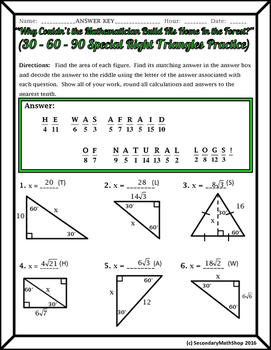



Right Triangles Special 30 60 90 Riddle Practice Worksheet Tpt



Math Figure 30 60 90 Triangle




30 60 90 Triangle Definition Theorem Formula Examples
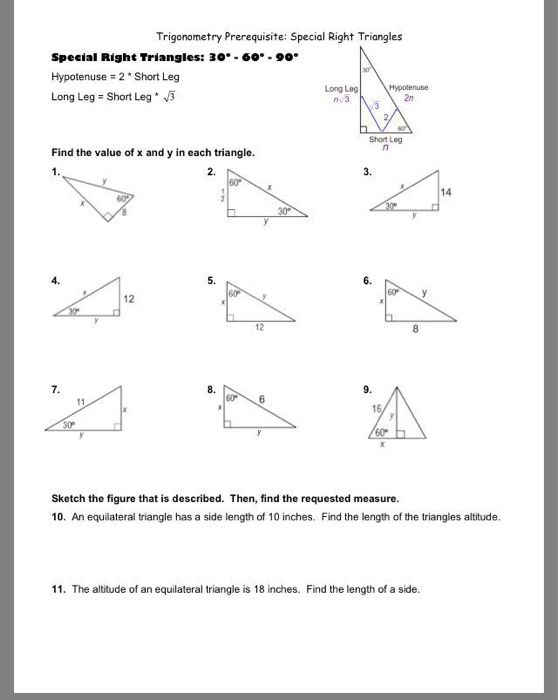



Solved Trigonometry Prerequisite Special Right Triangles Chegg Com




Ppt Triangles And Lines Special Right Triangles There Are Two Special Right Triangles Powerpoint Presentation Id



30 60 90 Special Right Triangles Videos Worksheets Examples Solutions




Special Right Triangles




Discovering Special Right Triangles 30 60 90 Triangles Made With Medians Of Equilateral Triangles Activity Builder By Desmos
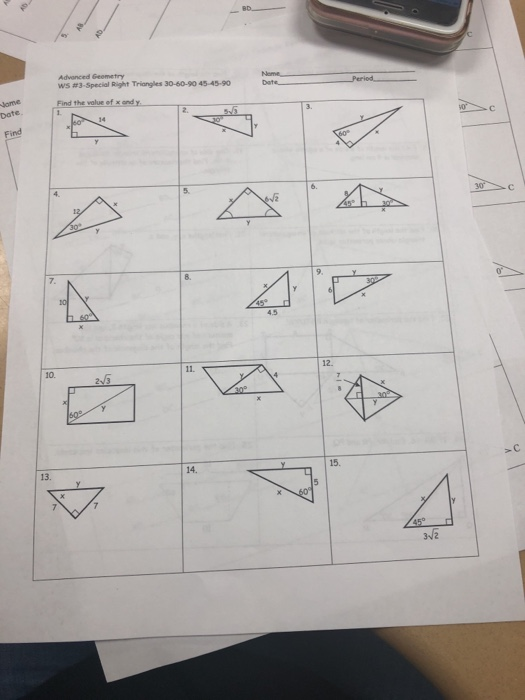



Solved Advanced Geemetry Ws 3 Special Right Triangles Chegg Com
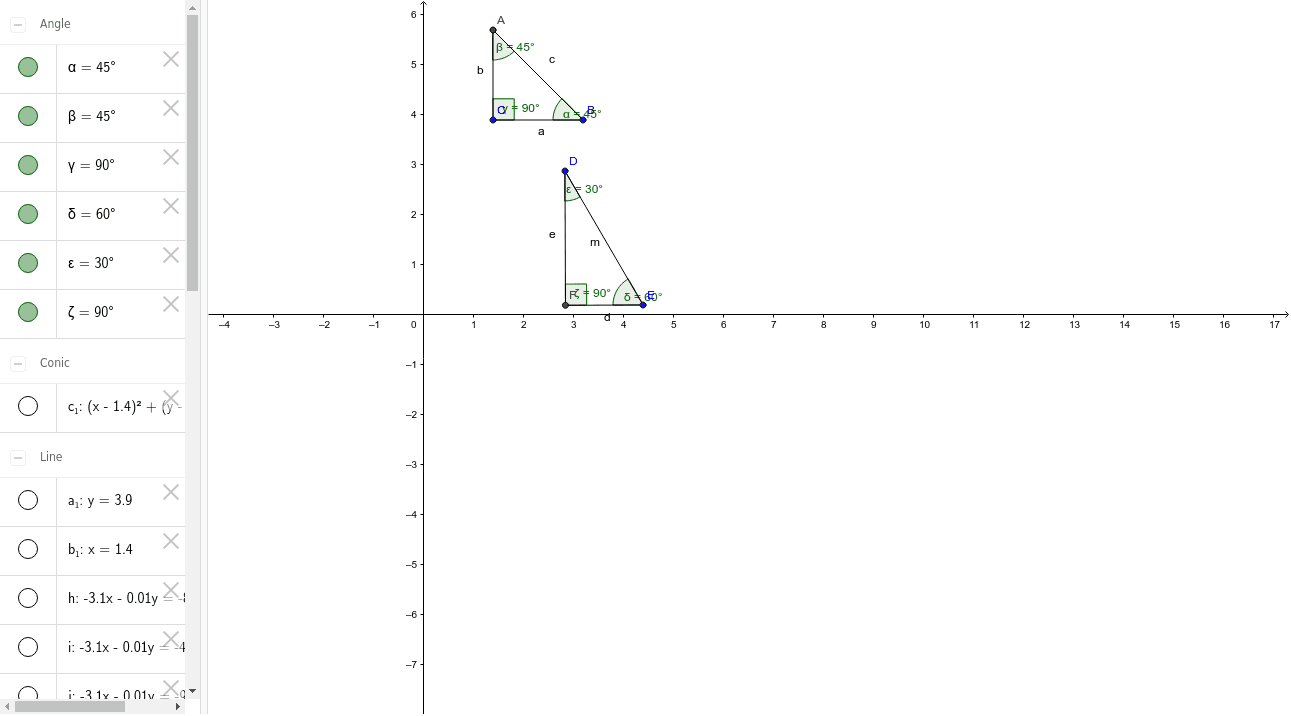



Special Right Triangles 45 45 90 Degree And 30 60 90 Geogebra



1
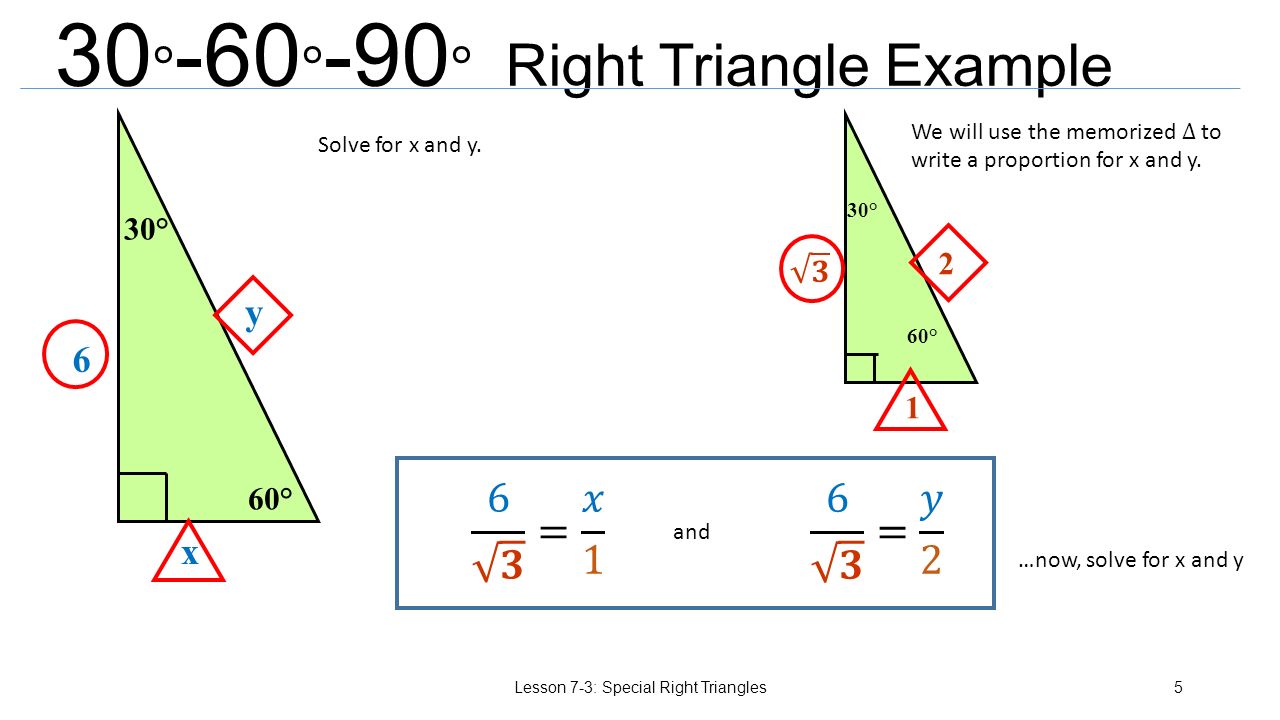



Special Right Triangles Lesson 7 3 Special Right Triangles1 Ppt Download




Right Triangles Interactive Notebook Pages Mrs E Teaches Math




Special Right Triangles 30 60 90 45 45 90 Flashcards Quizlet




30 60 90 Right Triangles Free Math Help




How To Work With 30 60 90 And 45 45 90 Degree Triangles Dummies




30 60 90 Triangle Theorem Ratio Formula Video



Special Right Triangle 30 60 45 45 37 53 Elearning




How To Use The Special Right Triangle 30 60 90 Studypug



Special Right Triangles Sss a Examples Included




A Quick Guide To The 30 60 90 Degree Triangle Dummies
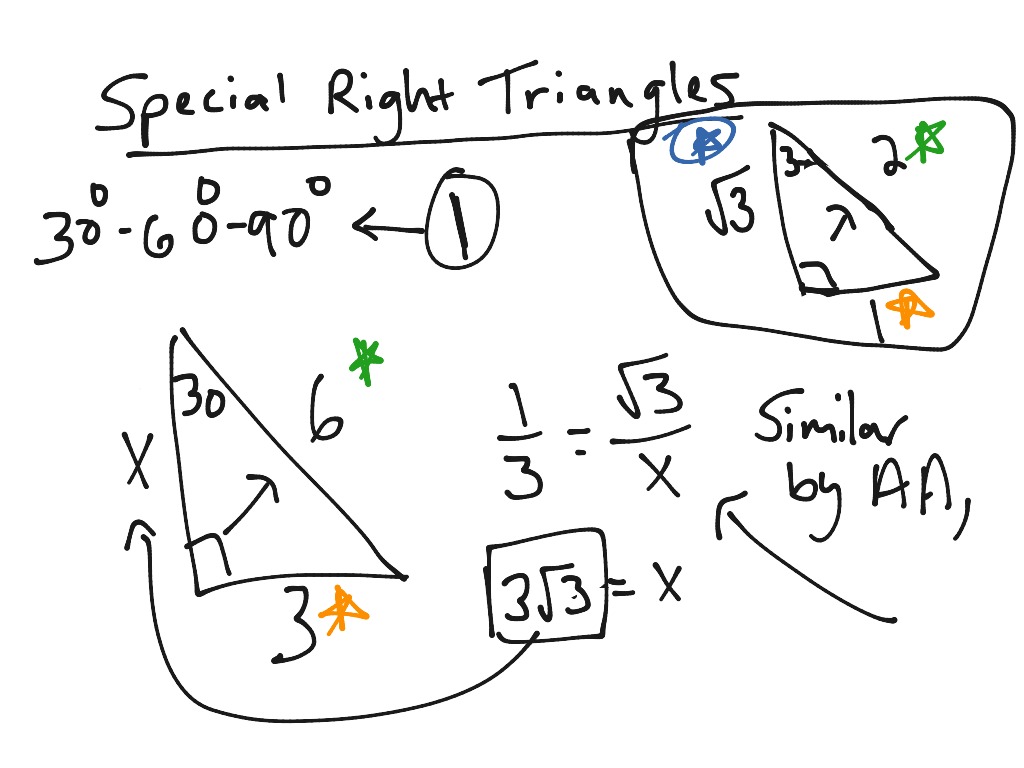



Special Right Triangles 30 60 90 Math Geometry Triangles Right Triangles Showme




Special Right Triangles Worksheet Geometrycoach Com




Special Right Triangles Gmatsyllabus Com



Geometry Lesson 7 2 Special Right Triangles Lessons Blendspace




45 45 90 Special Right Triangle Ppt Download




Special Right Triangles Review Article Khan Academy




Special Right Triangles Video Lessons Examples And Solutions
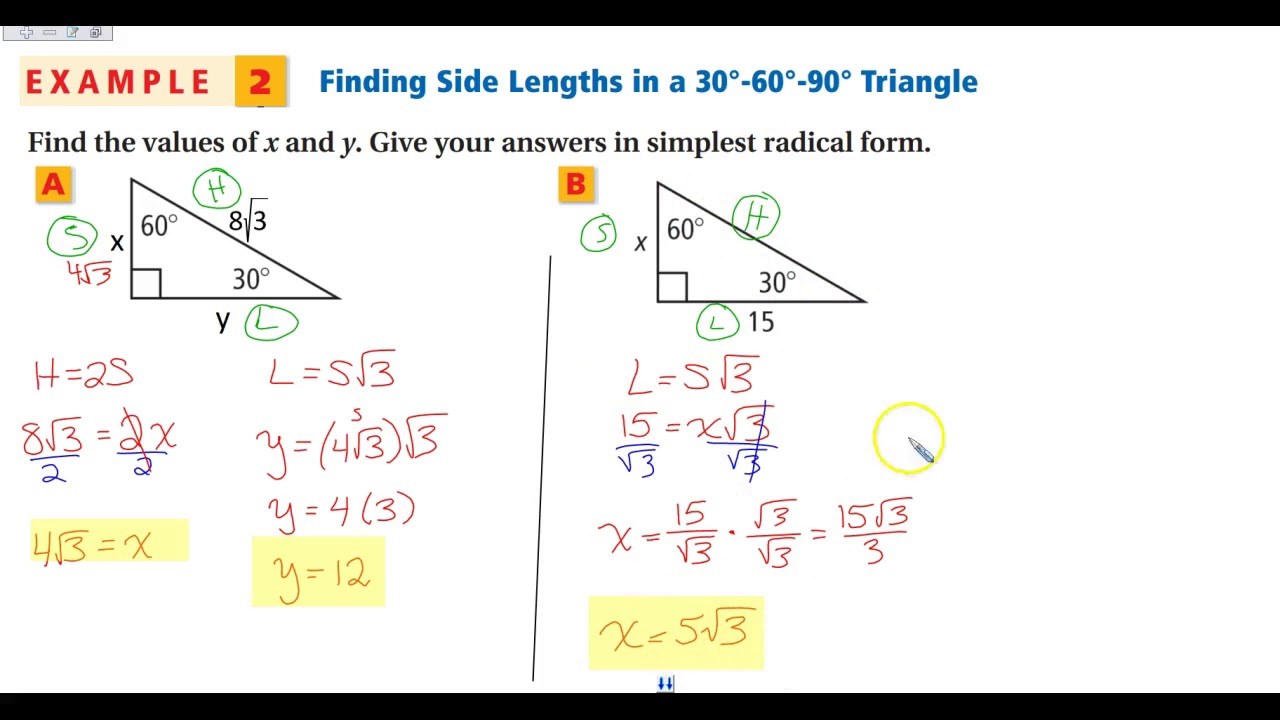



5 8b 30 60 90 Special Right Triangles Youtube




30 60 90 Triangles Spock S Guide To Math 4




The Converse Of The Pythagorean Theorem And Special Triangles Geometry Right Triangles And Trigonometry Mathplanet



The Easy Guide To The 30 60 90 Triangle




Special Right Triangles Fully Explained W 19 Examples




Math Special Right Triangles Exploring 30 60 90 Right Triangles Solve For X And Y In The Brainly Ph



Blog Posts Ferguson Loves Math
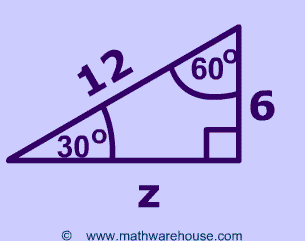



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems




30 60 90 Triangle Formulas Rules And Sides Science Trends
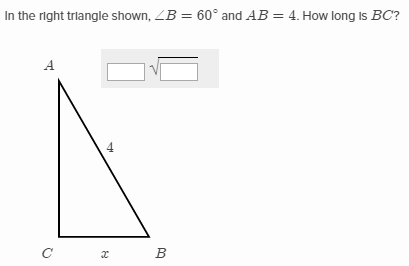



Special Right Triangles Khan Academy Wiki Fandom
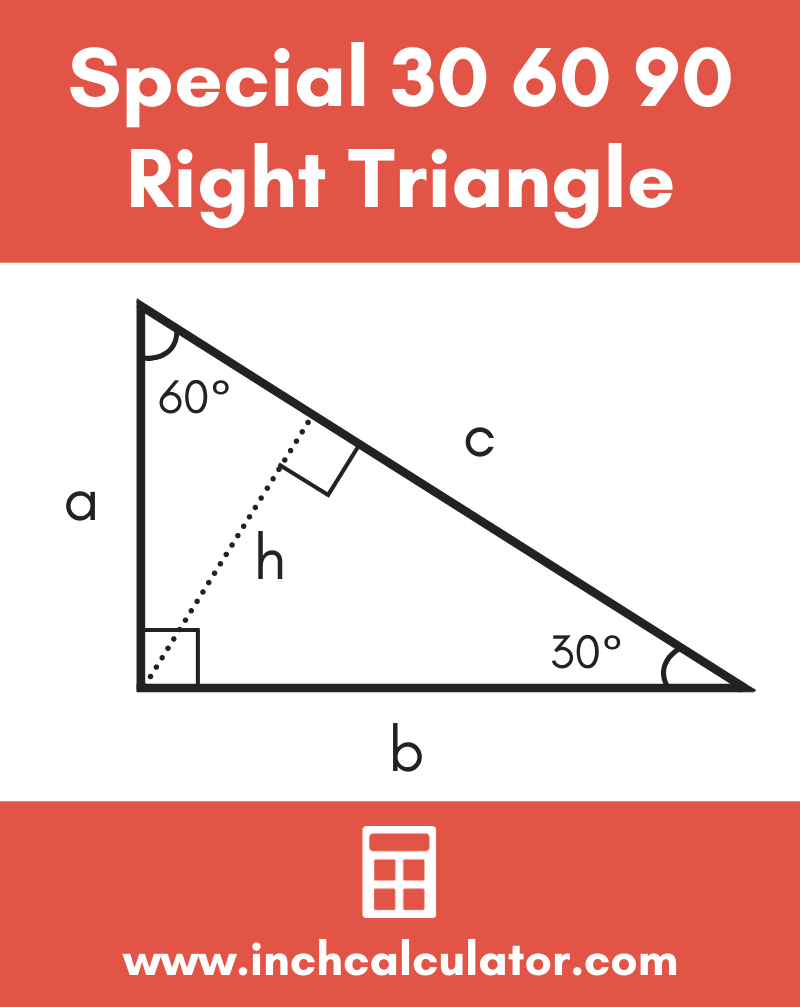



30 60 90 Special Right Triangle Calculator Inch Calculator
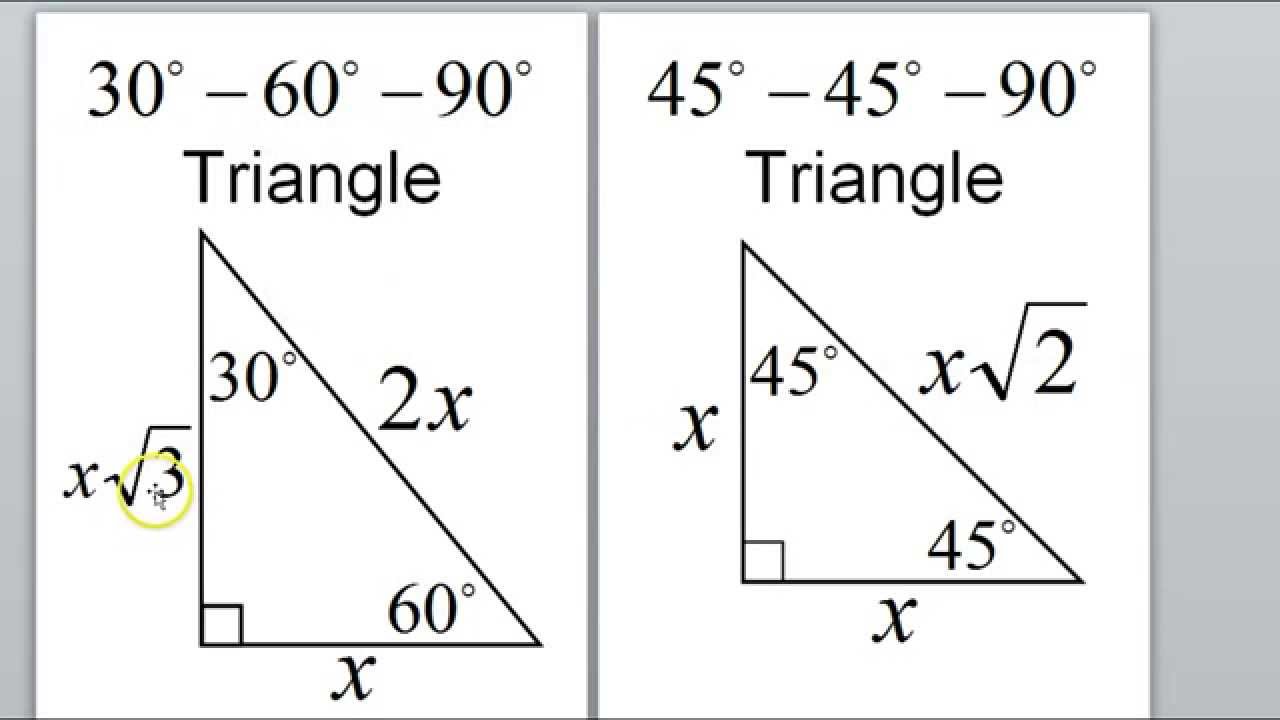



Day 1 Hw Special Right Triangles 45 45 90 30 60 90 Youtube
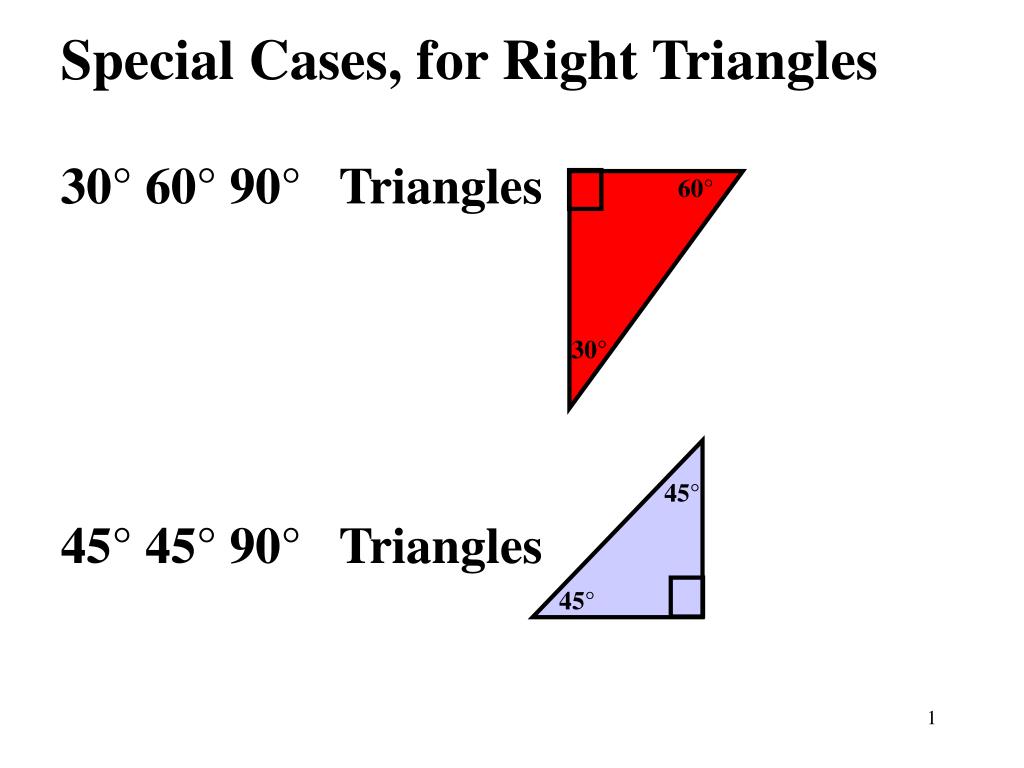



Ppt Special Cases For Right Triangles 30 60 90 Triangles Powerpoint Presentation Id




Special Right Triangles Interactive Notebook Page Math Methods Teaching Geometry Teaching Math
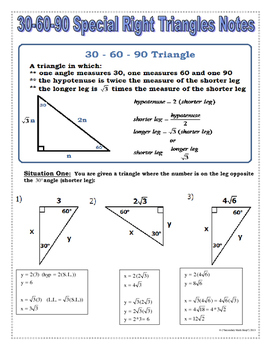



Right Triangles 30 60 90 Special Right Triangles Notes And Practice
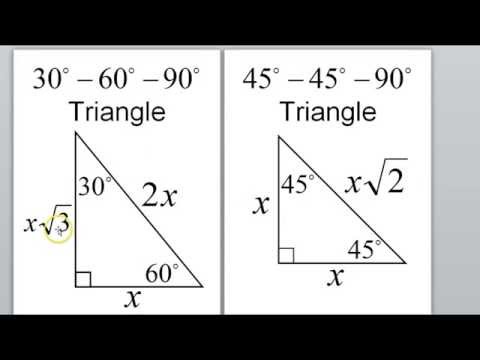



Special Right Triangles 30 60 90 Worksheet Jobs Ecityworks




Ideas And Resources For The Secondary Math Classroom Better Questions Special Right Triangles Teaching Geometry Math Geometry Activities Right Triangle




G 8 2 Special Right Triangles 1 4590
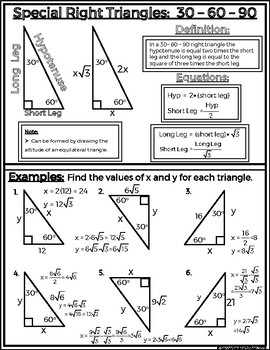



Special Right Triangles 45 45 30 60 90 Notes Sheet Graphic Organizers
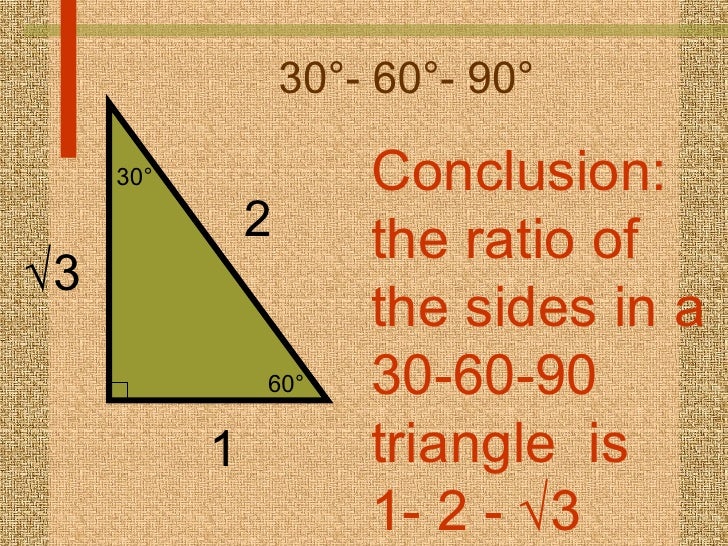



Special Right Triangles



The Easy Guide To The 30 60 90 Triangle
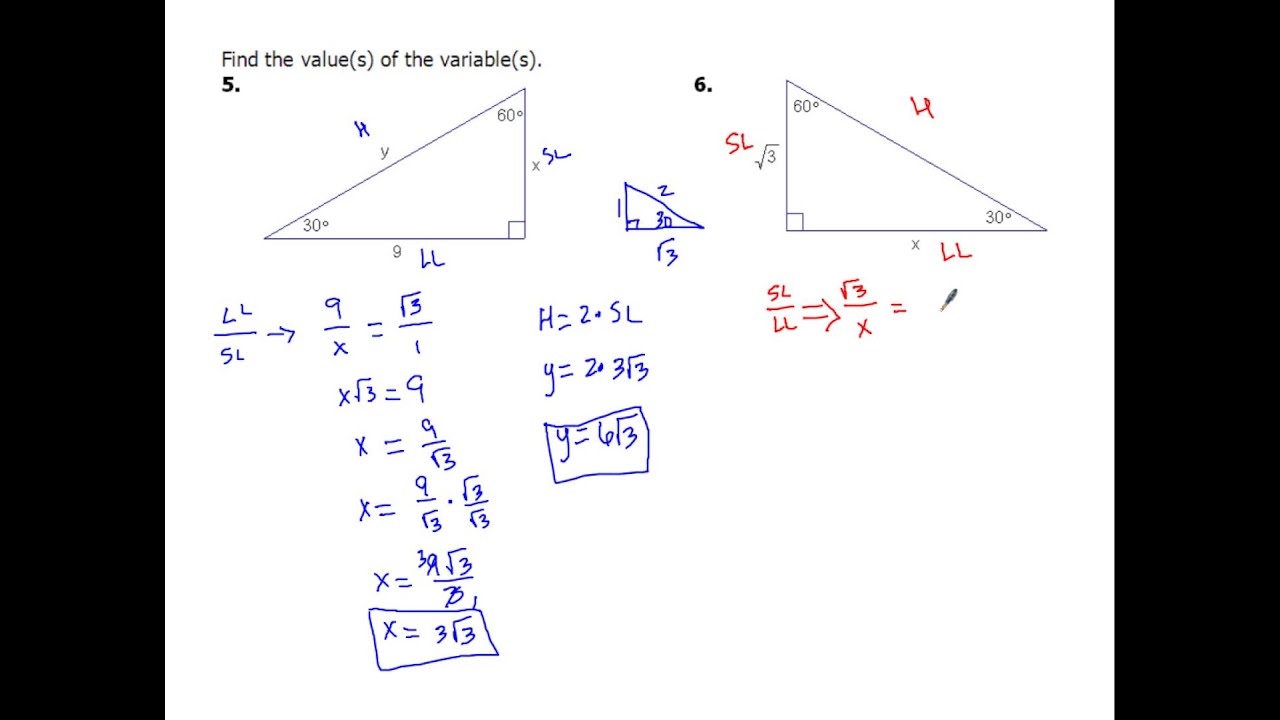



7 4d Solving 30 60 90 Special Right Triangles Youtube
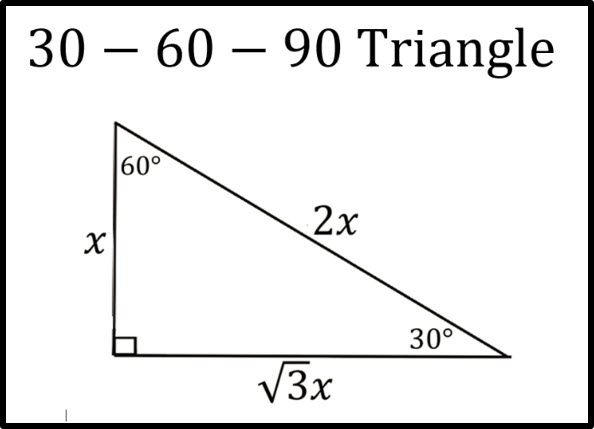



Special Right Triangles Andymath Com
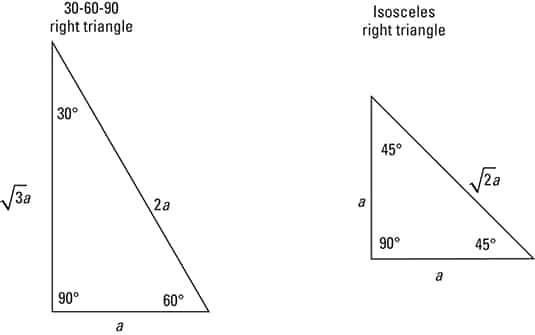



Understanding More About Special Right Triangles Get Education




Special Right Triangles 30 60 90 And 45 45 90 Quiz Quizizz
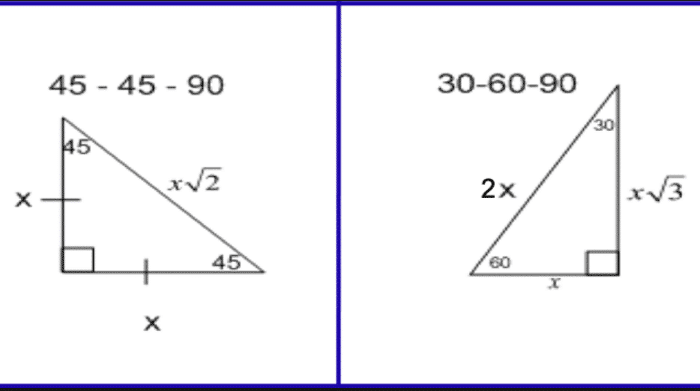



Special Right Triangles Complete Reference Guide The Education




30 60 90 Triangle Theorem Ratio Formula Video




Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math


